Introduction

Logarithms are a mathematical concept that plays a significant role in various fields, including mathematics, science, finance, and computer science. Understanding logarithms and their applications can help simplify complex calculations, solve exponential equations, and analyse data. In this article, we will explore the definition of logarithms, explain their properties, and highlight their practical applications in different domains.

Definition and Notation

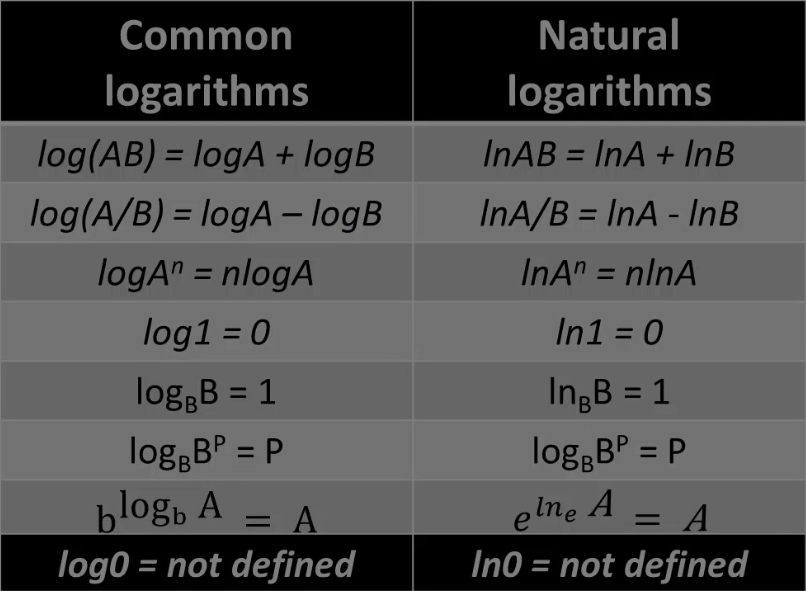
Logarithms are the inverse operation of exponentiation. The logarithm of a number to a specific base is the exponent to which the base must be raised to obtain that number. Mathematically, the logarithm of a number x with base b is denoted as log base b of x, or simply logₐ(x).

Properties of Logarithms

a) Product Rule: The logarithm of the product of two numbers is equal to the sum of their individual logarithms. Mathematically, logₐ(xy) = logₐ(x) + logₐ(y).

b) Quotient Rule: The logarithm of the quotient of two numbers is equal to the difference of their individual logarithms. Mathematically, logₐ(x/y) = logₐ(x) – logₐ(y).

c) Power Rule: The logarithm of a number raised to a power is equal to the product of the power and the logarithm of the number. Mathematically, logₐ(xⁿ) = n * logₐ(x).

Practical Applications of Logarithms

a) Data Analysis: Logarithms are commonly used in data analysis to compress a wide range of values into a more manageable scale. Logarithmic scales are useful when dealing with large numbers, such as in financial data, earthquake magnitude, or decibel levels. Logarithmic transformations can also help normalize skewed data distributions.

b) Exponential Growth and Decay: Logarithms are crucial in modelling exponential growth or decay. They are used to solve equations that involve exponential functions, such as population growth, radioactive decay, or compound interest calculations.

c) Signal Processing and Computer Science: Logarithms find applications in signal processing and computer science algorithms. For instance, they are used in audio compression algorithms (e.g., MP3) to reduce file sizes while maintaining perceptual quality. In computer science, logarithms are fundamental in analysing algorithm complexity and determining time and space efficiency.

d) Finance and Investment: Logarithms are utilized in finance and investment analysis, particularly in calculating returns and assessing risk. Logarithmic returns, such as compound annual growth rates (CAGR), help investors evaluate the performance of investments over time.

Conclusion

Logarithms are a powerful mathematical tool that simplifies calculations, solves exponential equations, and has diverse applications in various fields. Understanding the properties and applications of logarithms enables us to analyse data, model exponential phenomena, and make informed decisions in finance and science. By embracing logarithms, individuals can tackle complex problems efficiently, manipulate data effectively, and gain deeper insights into the world of numbers. Logarithms serve as a valuable asset in mathematics and its practical applications, paving the way for precise calculations and meaningful interpretations.